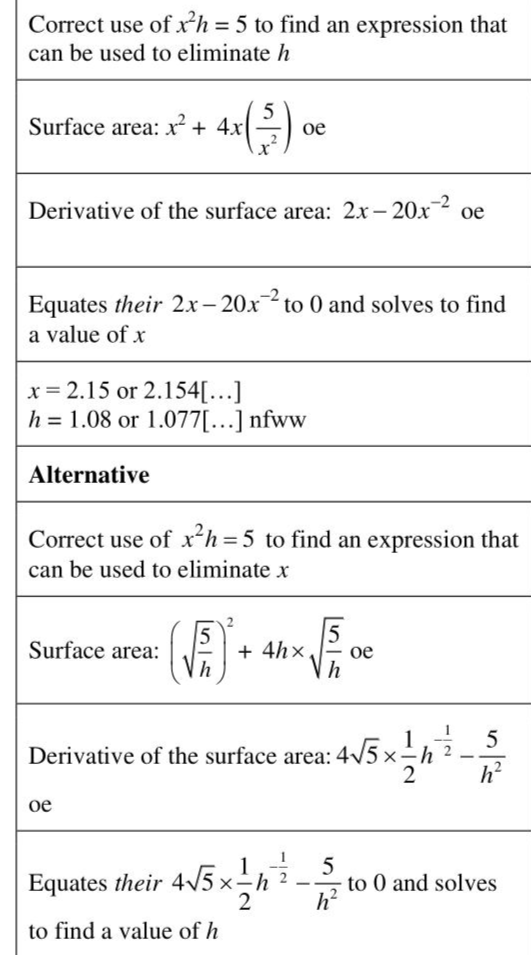
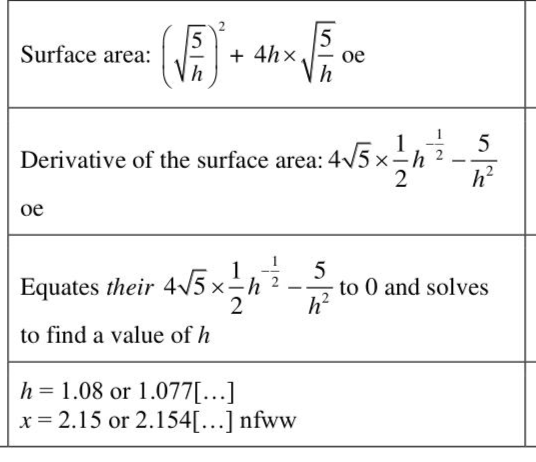
A metal tank is in the shape of a cuboid with a square base of side $$\(x \mathrm{~m}\)$$ and an open top. The tank has a volume of $$\(5 \mathrm{~m}^{3}\)$$. Given that $$\(x\)$$ can vary, and that the area of the metal used to make the tank is a minimum, find the dimensions of the tank. Cambridge Assessment International Education is part of Cambridge Assessment. Cambridge Assessment is the brand name of the University of Cambridge

Answer:

Knowledge points:

Solution: