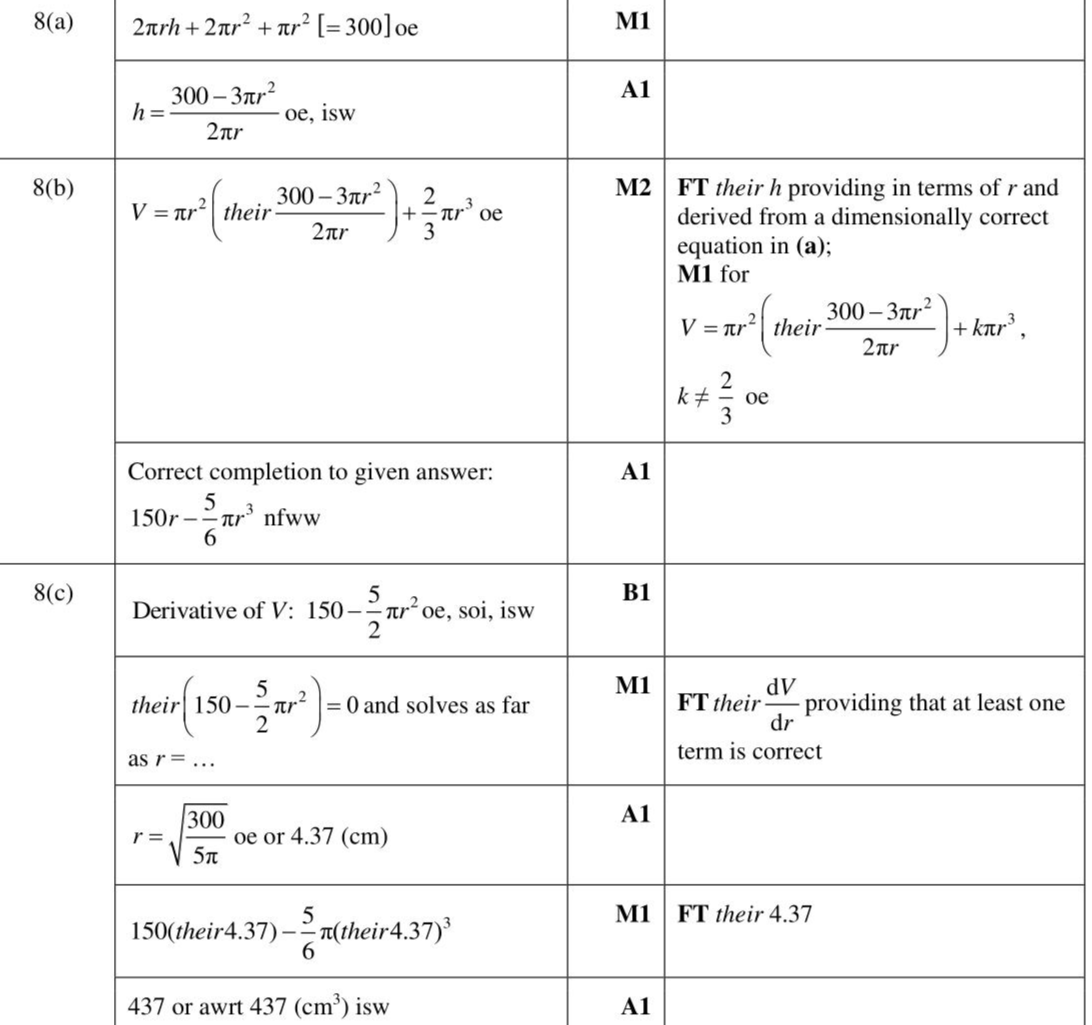
In this question all lengths are in centimetres. The volume of a cylinder with radius $$\(r\)$$ and height $$\(h\)$$ is $$\(\pi r^{2} h\)$$ and its curved surface area is $$\(2 \pi r h\)$$. The volume of a sphere with radius $$\(r\)$$ is $$\(\frac{4}{3} \pi r^{3}\)$$ and its surface area is $$\(4 \pi r^{2}\)$$. The diagram shows a solid object in the shape of a cylinder of base radius $$\(r\)$$ and height $$\(h\)$$, with a hemisphere of radius $$\(r\)$$ on top. The total surface area of the object is $$\(300 \mathrm{~cm}^{2}\)$$. (a) Find an expression for $$\(h\)$$ in terms of $$\(r\)$$. (b) Show that the volume, $$\(V\)$$, of the object is $$\(150 r-\frac{5}{6} \pi r^{3}\)$$. (c) Find the maximum volume of the object as $$\(r\)$$ varies.

Answer:

Knowledge points:

Solution: