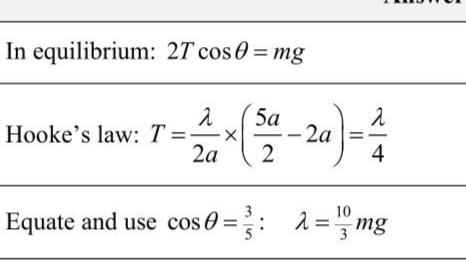
The points $$\(A\)$$ and $$\(B\)$$ are at the same horizontal level a distance $$\(4 a\)$$ apart. The ends of a light elastic string, of natural length $$\(4 a\)$$ and modulus of elasticity $$\(\lambda\)$$, are attached to $$\(A\)$$ and $$\(B\)$$. A particle $$\(P\)$$ of mass $$\(m\)$$ is attached to the midpoint of the string. The system is in equilibrium with $$\(P\)$$ at a distance $$\(\frac{3}{2} a\)$$ below $$\(M\)$$, the midpoint of $$\(A B\)$$. Find $$\(\lambda\)$$ in terms of $$\(m\)$$ and $$\(g\)$$. ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . ........................................................................................................................................................ . The particle $$\(P\)$$ is pulled down vertically and released from rest at a distance $$\(\frac{8}{3} a\)$$ below $$\(M\)$$.

Answer:

Knowledge points:

Solution: