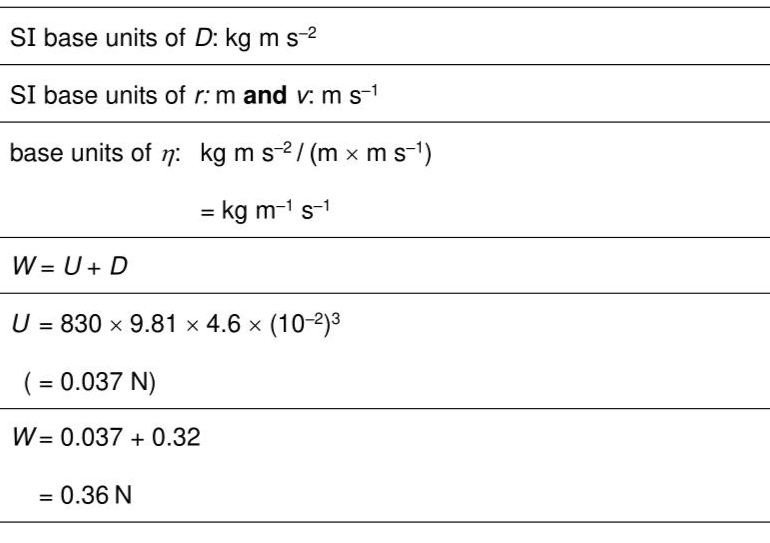
A sphere falls vertically through a liquid that has density $$$830 \mathrm{~kg} \mathrm{~m}^{-3}$$$. The sphere has radius $$$r$$$ and constant velocity $$$v$$$, as shown in Fig. 1.1. Fig. 1.1 (i) The drag force $$$D$$$ acting on the sphere is given by $$\[ D=6 \pi r \eta v \]$$ where $$$\eta$$$ is a property of the liquid. Determine the SI base units of $$$\eta$$$. SI base units ..................................................... (ii) State an equation showing the relationship between the magnitudes of the weight $$$W$$$, drag force $$$D$$$ and upthrust $$$U$$$ acting on the sphere. ................................................................................................................................. (iii) The volume of the sphere is $$$4.6 \mathrm{~cm}^{3}$$$. The drag force $$$D$$$ is 0.32 N . Calculate the weight of the sphere. weight = .................................................. N

Physics

IGCSE&ALevel

CAIE

Exam No：9702_w24_qp_22 Year：2024 Question No：1(b)

Answer:

Knowledge points:

1.2.1 recall the following SI base quantities and their units: mass (kg), length (m), time (s), current (A), temperature (K), amount of substance (mol)

1.2.2 express derived units as products or quotients of the SI base units and use the named units listed in this syllabus as appropriate

1.2.3 use SI base units to check the homogeneity of physical equations

1.2.4 use the following prefixes and their symbols to indicate decimal submultiples or multiples of both base and derived units: pico (p), nano (n), micro (μ), milli (m), centi (c), deci (d), kilo (k), mega (M), giga (G), tera (T)

1.2.5 understand and use the conventions for labelling graph axes and table columns as set out in the ASE publication Signs, Symbols and Systematics (The ASE Companion to 16–19 Science, 2000)

5.3.1 state and apply the principle of moments

5.3.2 understand that, when there is no resultant force and no resultant torque, a system is in equilibrium

5.3.3 use a vector triangle to represent coplanar forces in equilibrium

5.4.1 define and use density

5.4.2 define and use pressure

5.4.3 derive, from the definitions of pressure and density, the equation Δp = ρgDh

Answer:

Knowledge points:

Solution:

Related Question