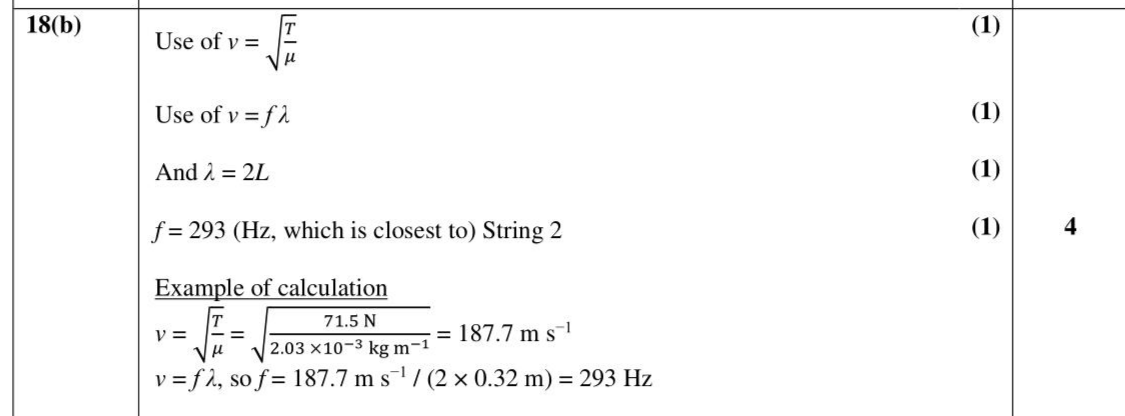
The photograph shows a musical instrument called a violin. The violin has four strings. Each string is held in a fixed position by a peg and at the bridge. When a string is plucked, a stationary wave forms on the string. The simplest stationary wave that can be formed on the string has a node $$\((\mathrm{N})\)$$ at each end and an antinode (A) at the centre, as shown. The frequency of this wave is called the fundamental frequency. The strings on a violin have different fundamental frequencies, as shown in the table. The tension in one of the strings is $$\(71.5 \mathrm{~N}\)$$. The length of the string is $$\(32 \mathrm{~cm}\)$$ and the mass per unit length of the string is $$\(2.03 \times 10^{-3} \mathrm{~kg} \mathrm{~m}^{-1}\)$$. Deduce whether this is string $$\(1,2,3\)$$ or 4 (4)

Answer:

Knowledge points:

Solution: